Admin
Admin
المساهمات : 93
تاريخ التسجيل : 21/09/2008
 |  موضوع: سلسلة محاضرات part1 strength of material  2008-09-28, 2:22 pm | |
|
2. Stresses
Stress is defined as the internal resistance set up by a body when it is deformed. It is measured in N/m2 and this unit is specifically called Pascal (Pa). A bigger unit of stress is the mega Pascal (MPa).
1 Pa = 1N/m2,
1MPa = 106 N/m2 =1N/mm2.
2.1. Three Basic Types of Stresses
Basically three different types of stresses can be identified. These are related to the nature of the deforming force applied on the body. That is, whether they are tensile, compressive or shearing.
|
| 2.1.1. Tensile Stress Consider a uniform bar of cross sectional area A subjected to an axial tensile force P. The stress at any section x-x normal to the line of action of the tensile force P is specifically called tensile stress pt . Since internal resistance R at x-x is equal to the applied force P, we have, pt = (internal resistance at x-x)/(resisting area at x-x) =R/A =P/A. Under tensile stress the bar suffers stretching or elongation. | | | | | <table cellSpacing=0 cellPadding=0 width=336 border=0><tr><td vAlign=top>
window.google_render_ad();
</TD></TR></TABLE> | | | 2.1.2. Compressive StressIf the bar is subjected to axial compression instead of axial tension, the stress developed at x-x is specifically called compressive stress pc. pc =R/A = P/A. 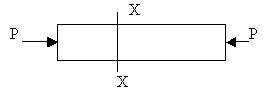 Under compressive stress the bar suffers shortening. | | | 2.1.3. Shear Stress
Consider the section x-x of the rivet forming joint between two plates subjected to a tensile force P as shown in figure. | | | The stresses set up at the section x-x acts along the surface of the section, that is, along a direction tangential to the section. It is specifically called shear or tangential stress at the section and is denoted by q.
q =R/A
=P/A. | | | 2.2. Normal or Direct Stresses
When the stress acts at a section or normal to the plane of the section, it is called a normal stress or a direct stress. It is a term used to mean both the tensile stress and the compressive stress.
2.3. Simple and Pure Stresses
The three basic types of stresses are tensile, compressive and shear stresses. The stress developed in a body is said to be simple tension, simple compression and simple shear when the stress induced in the body is (a) single and (b) uniform. If the condition (a) alone is satisfied, the stress is called pure tension or pure compression or pure shear, as the case may be.
2.4. Volumetric Stress
Three mutually perpendicular like direct stresses of same intensity produced in a body constitute a volumetric stress. For example consider a body in the shape of a cube subjected equal normal pushes on all its six faces. It is now subjected to equal compressive stresses p in all the three mutually perpendicular directions. The body is now said to be subjected to a volumetric compressive stress p. <table cellSpacing=0 cellPadding=0 width="90%" align=center border=0><tr><td vAlign=top> 3. Strains
Strain is defined a the ratio of change in dimension to original dimension of a body when it is deformed. It is a dimensionless quantity as it is a ratio between two quantities of same dimension.</TD></TR> <tr><td vAlign=top> </TD></TR> <tr><td vAlign=top> 3.1. Linear Strain
Linear strain of a deformed body is defined as the ratio of the change in length of the body due to the deformation to its original length in the direction of the force. If l is the original length and dl the change in length occurred due to the deformation, the linear strain e induced is given by e=dl/l.
 Linear strain may be a tensile strain, et or a compressive strain ec according as dl refers to an increase in length or a decrease in length of the body. If we consider one of these as +ve then the other should be considered as –ve, as these are opposite in nature. </TD></TR> <tr><td vAlign=top> </TD></TR> <tr><td vAlign=top> 3.2. Lateral Strain
Lateral strain of a deformed body is defined as the ratio of the change in length (breadth of a rectangular bar or diameter of a circular bar) of the body due to the deformation to its original length (breadth of a rectangular bar or diameter of a circular bar) in the direction perpendicular to the force. </TD></TR> <tr><td vAlign=top> </TD></TR> <tr><td vAlign=top> <table cellSpacing=0 cellPadding=0 width=336 border=0><tr><td vAlign=top>
window.google_render_ad();
</TD></TR></TABLE> </TD></TR> <tr><td vAlign=top> </TD></TR> <tr><td vAlign=top> 3.3. Volumetric StrainVolumetric strain of a deformed body is defined as the ratio of the change in volume of the body to the deformation to its original volume. If V is the original volum and dV the change in volume occurred due to the deformation, the volumetric strain ev induced is given by ev =dV/V
Consider a uniform rectangular bar of length l, breadth b and depth d as shown in figure. Its volume V is given by,  This means that volumetric strain of a deformed body is the sum of the linear strains in three mutually perpendicular directions. </TD></TR> <tr><td vAlign=top> </TD></TR> <tr><td vAlign=top> 3.4. Shear Strain
Shear strain is defined as the strain accompanying a shearing action. It is the angle in radian measure through which the body gets distorted when subjected to an external shearing action. It is denoted by *.
 Consider a cube ABCD subjected to equal and opposite forces Q across the top and bottom forces AB and CD. If the bottom face is taken fixed, the cube gets distorted through angle * to the shape ABC’D’. Now strain or deformation per unit length is
Shear strain of cube = CC’ / CD = CC’ / BC = * radian</TD></TR> <tr><td vAlign=top> </TD></TR></TABLE> |
| |
|
