Admin
Admin
المساهمات : 93
تاريخ التسجيل : 21/09/2008
 |  موضوع: 3- تقرير عن center of pressure موضوع: 3- تقرير عن center of pressure  2008-09-23, 7:06 pm 2008-09-23, 7:06 pm | |
| Centre of pressure on a plane Surface HOMEPAGE
A fabricated quadrant is mounted on a balance arm, which pivots on knife edges. The line of contact of the knife edges coincides with the axis of the quadrant. Thus, of the hydrostatic forces acting on the quadrant when immersed, only the force on the rectangular face end gives rise to a moment about the knife edge axis.
In addition to the quadrant clamping screw the balance arm incorporates a balance pan, an adjustable counterbalance and an indicator which shows when the arm is horizontal.
The Perspex tank should be levelled by adjusting the screwed feet. Correct alignment is indicated by a circular spirit level mounted on the base of the tank.
Water is admitted to the top of the tank by a flexible tube and may be drained through the drain cock in the base. The water supply is obtained from the hydrostatic work bench. The water level in the Perspex tank is indicated on a scale.
Objectives
To determine the hydrostatic thrust acting on a plane surface immersed in water.
To determine the position of the line of action of the thrust and to compare the position determined by experiment with the theoretical position.
Method
By achieving an equilibrium condition between the moments acting on the balance arm of the test apparatus. The forces concerned are the weight force ‘W’ applied to the balance and the hydrostatic pressure thrust ‘F’ on the end face of the quadrant.
Technical Data
The following dimensions from the equipment are used in the appropriate calculations. These values should be checked as part of your experimental procedure and replaced with your own measurements.
Length of Balance L 275 mm Distance from weight hanger to pivot
Quadrant to Pivot H 200 mm Base of quadrant face to pivot height
Height of Quadrant D 100 mm Height of vertical quadrant face
Width of Quadrant B 75 mm Width of vertical quadrant face
Apparatus
The Armfield Hydrostatic Pressure Apparatus, F1-12

Procedure - Equipment Calibration
Measure the dimensions B and D of the quadrant end-face and the distances H and L. Update the values in your results table as necessary.
Procedure - Equipment Set Up
Position the empty F1-12 tank on the hydrostatic bench, and adjust the screwed feet until the built-in circular spirit level indicates that the base is horizontal. Position the balance arm on the knife edges. Locate the weight hangar in the groove at the end of the balance arm.
Ensure that the drain valve is closed and that a length of clear plastic pipe is attached to the drain cock. Direct the free end of this pipe to the sink in the bench. Take the free end of the delivery pipe which supplies water to the other apparatus on the bench and place it in the triangular aperture on top of the plastic tank.
Move the counter-balance weight until the balance arm is horizontal.
Procedure - Taking a Set of Results
Add a small mass (50g) to the weight hanger.
Add water until the hydrostatic thrust on the end-face of the quadrant causes the balance arm to raise. Ensure that there is no water spilled on the upper surfaces of the quadrant or the sides, above the water level.
Continue to add water until the balance arm is horizontal, measuring this by aligning the base of the balance arm with the top or bottom of the central marking on the balance rest (either can be used, but it must be kept consistent during the experiment). You may find it easier to slightly over-fill the tank, and obtain the equilibrium position by opening the drain cock to allow a small outflow.
Read the depth of immersion from the scale on the face of the quadrant; more accurate results can be obtained from reading with the line of sight slightly below the surface, to avoid the effects of surface tension.
Repeat the above procedure for each load increment, produced by adding a further weight to the weight hanger. The weights supplied allow increments of ten, twenty, and fifty grams to be used, depending on the number of samples required. Fifty-gram intervals are suggested for an initial set of results, which will give a total of nineteen samples.
Continue until the water level reaches the top of the upper scale on the quadrant face. Repeat the procedure in reverse, by progressively removing the weights.
Note any factors that you think are likely to affect the accuracy of your results.
Results
Your raw data should be presented in a table using the following headings:
Mass, m (gm) Depth of immersion, d (mm)
Using the equations from the theory below, calculate the Hydrostatic Thrust F then calculate the experimental and theoretical position of the centre of pressure P, relative to the pivot, from your experimental results. Note that there will be different results for the case when the vertical plane is partly submerged and fully submerged.
Your results should be tabulated as follows:
Thrust, F (N)
Depth of Centre of Pressure, h" experimental (mm)
Depth of Centre of Pressure, h" theoretical (mm)
Now plot graphs of the thrust against depth of immersion and the depth of the centre of pressure against the depth of immersion.
Conclusions
1. Comment on the variation of thrust with depth.
2. Comment on the relationship between the depth of the centre of pressure and the depth of immersion.
For both (1) and (2) above, comment on what happens when the plane has become fully submerged.
Comment on and explain any discrepancies between the experimental and theoretical results for the depth of centre of pressure.
Theory section
PRESSURE ON A SURFACE IMMERSED IN A LIQUID
Whilst the basic theory for the partly submerged and fully submerged plane is the same, it will be clearer to consider the 2 cases separately.
1. Partly submerged vertical plane surface

Thrust on surface:
Hydrostatic thrust,  (Newtons) (Newtons)
Where A = B x d and d = depth of immersion. See figure above.
And  = depth of the centroid of the submerged area C, = = depth of the centroid of the submerged area C, = 
Thus  …………………. Eqn 1 …………………. Eqn 1
Moment of thrust about pivot
Moment M = F x h” (Nm)
Where h” = depth of line of action of thrust below pivot. I.e. centre of pressure P.
Equilibrium condition
A balancing moment is produced by the weight (W) applied to the hanger at the end of the balance arm = W x L (Nm).
For static equilibrium the two moments are equal.
i.e. F x h” = W x L = m x g x L (m = applied mass)
Thus h” =  = =  (metres) …….Eqn 2 (metres) …….Eqn 2
Remember,
 so h” = so h” =  , the gravity terms cancel, leaving you with Eqn 2 , the gravity terms cancel, leaving you with Eqn 2
The theoretical result for depth of centre of pressure, P, below the free-surface of the fluid is:
h’ =  …….Eqn 3 …….Eqn 3
where Ix = 2nd moment of area of immersed section about an axis in the free water surface.
So  (using the parallel axis theorem) (using the parallel axis theorem)
Thus  which is = which is =  (m4) …….Eqn 4 (m4) …….Eqn 4
The depth of ‘P’ below the pivot point will be:
h” = h’ + H - d (m)
and if you substitute Eqn 4 into equation 3 you will get :- h” = H - d/3
as the theoretical result.
In other words, the distance from the pivot to the centre of pressure is the depth to the bottom of the vertical plane, minus one third the depth of the submerged part of the vertical plane. So the centre of pressure on a partially submerged plane will always be one third of d up from the base of the plane surface.
However, when the plane is fully submerged, you now have to include the additional depth from the free water surface to the top of the vertical plane.
2. Fully submerged vertical plane surface

Though it is not shown in the diagram above, the pivot point still lies on the top horizontal solid line.
Thrust on surface:
Hydrostatic thrust,   (Newtons) (Newtons)
Where A = B x D and D = depth of vertical surface. See figure above.
And  = depth of the centroid of the submerged area C, = = depth of the centroid of the submerged area C, = 
Thus, 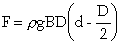 (Newtons) (Newtons)
Moment of thrust about pivot
Moment M = F x h” (Nm) As in previous part.
Equilibrium condition
As before, a balancing moment is produced by the weight (W) applied to the hanger at the end of the balance arm = W x L (Nm).
For static equilibrium the two moments are equal.
i.e. F x h” = W x L = m x g x L (m = applied mass)
But this time, h” =  = = 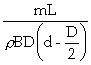 (metres) (metres)
The theoretical result for depth of centre of pressure, P, below the free-surface of the fluid is:
h’ = 
where Ix = 2nd moment of area of immersed section about an axis in the free water surface.
Thus:  (using the parallel axis theorem) (using the parallel axis theorem)
Thus  (m4) (m4)
So, the depth of ‘P’ below the pivot point will be:
h” = h’ + H - d (m) but it does not simplify as before.
|
| |
|
